Part 7 - Bearing stiffeners

And now for the really scary bits (it took 8 years for me to find this stuff out) 
As I mentioned earlier, the theory of U-frame restraint relies on effective bearing stiffeners, and needs to be modified if the bearing stiffeners are not up to the job. In order to see if they are strong enough, we obviously need some loads to check against. For reasons best known to the chaps with pointy heads, who wrote the code originally, the loads are split into two separate sections of the code, with an extra section between to confuse.

Just to be controversial, and it will be interesting to see if you remember this once you wade through the following sections, I believe that some of the loads in the code are a fairy tale and overestimate the effects significantly. I also am having problems finding one significant load that should be in there but does not appear to be.
9.14.3.1 a) reaction plus vertical component from bottom flange

Quite reasonably, the bearing stiffener sits on the bearing and has to transmit all of the vertical reaction into that bearing. If the bottom flange is at an angle, you are also required to include the component from the flange: this is odd. The reaction at the end is whatever it is and does not depend on how the load gets into it. If the beam is simply supported then it does not matter what angle the flange comes in at since there is no stress and hence no force. If there is an internal bearing with two flanges coming down at an angle then these will have vertical components which will, if anything, bypass the bearing stiffener and reduce the force in the stiffener below the reaction force.

Overall, I am a little wary about the reaction load since stiffeners are not only checked at the bottom but, according to 9.14.4, analysed all the way up the stiffener. The shear coming along the beam will gradually increase the force from close to zero near the top (apart from tension field action and sloping flanges in continuous beams) so it is quite conservative to apply the same load all the way up: unless doing something special, you have to follow the code blindly though.
9.14.3.2 axial force representing the destabilising influence of the web - meh

This, again, is a fiction, where a bending stress is replaced by an axial force: it only applies to internal supports, not to simply supported ends.

9.14.3.1 c) axial force due to transfer of load through cross frame or beam

Similar comments would apply as for the web stiffener. However, if the cross beam is close to the bottom then you should consider whether or not to calculate the load path separately (this will often be very short) and ignore any effect on the rest of the stiffener above the affected area.

9.14.3.1 d) various bending moments

This is a whole can of worms. I can totally understand how a cross girder (possibly a trimmer beam) could try to turn the stiffener over, and it should be assumed to be fixed ended to work out how much moment it will transfer into the bearing stiffener. I can even see how some eccentricity should be included, side to side, since the bearing might not sit centrally.

I cannot, however, get my head around eccentricity along the line of the beam. The effective stiffener includes a small length of web plate along the beam, in accordance with 9.14.2 which results in, typically, a bit of web plate with plates sticking out sideways forming a cruciform or similar. But if the bearing is assumed to sit forwards of the stiffener centroid by, say, 100mm due to temperature, you can get some massive theoretical moments applied to a notional cantilever member on the assumption that it is acting in isolation. But what will happen in practice is that cantilever is supported by the longitudinal stresses in the web and top flange. This argument also applies to f). It is definitely worth considering that a stiffener with two pairs of stiffener plates might move so far that one plate moves out of the zone of influence and then applying the load to a smaller stiffener, but assuming that the stiffener bends as a cantilever is rather odd.
9.14.3.1 e) leading to 9.12.5.2 – the fun bits

Now we get down to the nitty gritty. 9.12.5.2 includes four little darlings, referred to as F_S1 to F_S4. If I get bored at meetings, or if someone gets too full of themselves (a fault that I, of course, never suffer from) I enjoy steering the questions around to the derivation of these forces. No-one had ever given me a sensible explanation, so I recently took the opportunity, when doing some higher level analysis, to check out the first two from first principles. I have never had to worry about the last two factors and will continue with that approach here.

F_S1 – force due to bow of compression flange (the easy bit)

This force has a very non-linear equation which requires careful handling. 0.5% of the bending moment at midspan is divided by the height of the top flange above either the top of the cross girder (for U-frame) or the bottom flange (if not restrained by a cross girder) to give a transverse force at the top flange level. This force is increased by a VERY NON-LINEAR factor of 1/(1 – (σ_fc/(σ_ci)²), where σ_fc is the maximum top flange stress in the span and σ_ci is the top flange buckling stress. This would appear to be another hand waving magnification of a basic effect, and is as good as anything else.

Hopefully you will already have worked out that this cannot be treated separately for dead and live load, but has to be treated for total effects. You should be able to see that the non-linear can only be less than one and will approach zero as the bending stress approaches the buckling stress: dividing by zero tends to give very big numbers. If you calculate the effect past the buckling load, you will get an answer, but it would be meaningless (and negative) since a buckled beam cannot carry any load.

F_S1 – force due to bow of compression flange (more difficult bit)

Please bear in mind that the commentary from the SCI is exactly that, a commentary on what someone else has decided, and please do not take any of my snarky comments as a comment about the competence of the SCI, whose commentary is well worth buying.

The commentary states “The force F_S1 represents the end torque due to an initial sinusoidal bow of the compression flange for a simply supported beam under uniform moment”. The code multiplies the Part 6 construction tolerance, of length / 1000, by 1.5 so that the imperfection of the top flange, Δ_0, is assumed to be L/667.
Using a sinusoidal profile on plan:

y = Δ₀.sin{(𝜋/2)/(L/2).x} = Δ₀.sin{(𝜋/L).x}

dy/dx = (Δ₀. 𝜋/L)cos{(𝜋/L).x} = Δ₀. 𝜋/L at x=0 i.e. at beam end

Δ₀. 𝜋/L is therefore just the end “slope” of the beam on plan.

Δ₀ = L . 1.5 / 1000 so end slope = 𝜋 . 1.5 / 1000 = 0.0047 cf 0.005 in code.

Please note that if a parabolic profile were assumed (more sensible in my opinion since the constant curvature is more likely for a fabrication error) then the slope would be 27% higher at around 0.006.
The term 0.005 is therefore merely the component of the uniform moment (applied in the plane of the deformed web) that acts about a line joining the two support points.
The non-linear bit below is a multiplier that increases the initial imperfection due to P.delta effects as the load in the beam reaches buckling loads and the flange kicks sideways.
Easy !!
BUT, and yet again, you knew there was going to be one …

Please, however, cast your mind back to the initial statement of “for a simply supported beam under uniform moment”. Under what circumstances does a simply supported beam have a uniform moment along its length ? This is only possible if you hang some moment applying contraption at each end, and remove all other load in between. A real beam is more likely to have loads that approximate to UDL, giving a parabolic moment pattern and zero end moment. Even for a continuous beam you could just work out the moments on either side and take their components: it is a shame that they would cancel out giving a total of zero.

Rant: In my humble opinion (no laughing at the back if you actually know me) the calculation is total eyewash (oh the restraint required to write something I would be happy for my Mother to read). It does, however, give a higher load than other effects, not included anywhere.
This clause is applied to all beams, regardless of how they are configured. In most of my work, the loads are applied to the bottom of the main girder, so you could approximate the loads as being applied along the line where the main girder web and bottom flange meet.
If the bottom flange were deformed, then if you look along the line joining the bearings, then there would be a small transverse eccentricity of the applied loads which, when you consider the beam as a closed system, might cause some overturning moment at the boundary (i.e. the supports). But in most cases that I look at, the bottom flange is going to be held in place by plan bracing (or U-frame effects would not be very effective) so the bottom flange will be held roughly in place. You may, therefore, get some of the moment, but it will not be amplified by buckling. You will also get some end moment from end rotations of cross girders, if they are moment connected to the main girder: again, there will not be an amplification.
If you have some load, such as wet concrete on top of a composite beam during construction, then you could have some out of balance moments due to eccentricity, increasing as you approach buckling, but these are usually going to be braced against lateral torsional buckling, and if they are not then the bearing stiffener is likely to be the least of the problems.
F_S2 force due to non-verticality of web at supports (the easy-ish bits)

This one is a real beauty and demonstrates everything that is wrong with BS 5400 as a document for working engineers. Part of my recent high-level analysis involved dissecting the calculations produced by another firm of Consultants, who are well respected and not at all daft, but who made a few errors (as we all do).

The bearing stiffeners on this railway bridge were massively overloaded, and only appeared able to carry about half of the required load: most of this overloading came from F_S2.

Please, please tell me that you have spotted that this is non-linear. Unfortunately, the assessor did not and with values of  σ_ci = 175, which is the stress at which the beam will buckle (but see later !) and σ_fc = 25 for dead load and 100 for live load there was a large error because the items were calculated separately. The dead load effect was divided by 175 – 25, and the live load by 175 – 100, whereas they should both have been divided by 175 – 125: overall the already high loads were increased by around 2/3. The total load was corrected from 500 to 825kN.

But when I analysed this problematic beam using a geometrically non-linear analysis, I obtained a plot of the vertical stresses in the bearing stiffener, and these were, for all intents and purposes, symmetric, indicating that there were no transverse moments on the bearing stiffener. I therefore ran the problem through our company spreadsheet and found that the answer should have been 2kN, reducing the force by a factor of more than 400! WTF !!!
This is where BS 5400 really annoys me and I can totally see why someone who wrote their own spreadsheet from scratch could make this mistake. The equation for F_S1 also refers to the two stresses,  σ_ci and σ_fc , and these clauses are about an inch apart on the printed page (if you are too young to remember inches, refer to Wikipedia). There are also almost identical lines at the bottom of each clause that include “where … σ_ci, σ_fc  … are as defined in 9.12.5.2.5”.
If you go to clause 9.12.5.2.5, (more than a page away) you will find a very nasty sting in the tail.
“σ_ci is as defined in 9.12.2. In determining σ_ci for use in calculating F_S1, l_e should be derived in accordance with 9.6.2 for beams without intermediate restraints or 9.6.4 for beams with intermediate restraints. In determining σ_ci for use in calculating F_S2, l_e should be derived from 9.6.4.1.1.2b) for simply supported beams or 9.6.2b) for continuous beams”.
Nice.
This looks quite innocent but typically, the effective length for F_S1 is likely to be a reasonable proportion of the total span length, such as a half with reasonable U-frames, but the full length without U-frames, as was the case here. The effective length for F_S2 is a completely different number, is actually nothing to do with strut buckling, and is more likely to be around 10% of the span length. Since the “Buckling” stress (which is a complete misnomer here, as I will demonstrate if you can cope with the complicated next section) depends on 1/length², the buckling stress increased by a factor of 110 (175 MPa increased to 20,000MPa) and the force reduced by a factor of 400.

Rant: BS 5400 is written rigorously, and there is no room for misinterpretation IF YOU READ EVERY CLAUSE RELIGIOUSLY AND TAKE NOTHING FOR GRANTED: it is a shame that we engineers are human and this should never have got through into the document.

The only reason why I did not get caught was because I, and one of my colleagues, have been developing a set of modern analysis spreadsheets based on work produced by some of my colleagues a decade ago, working closely with a knowledgeable Client, and someone included for this terrible bit of code drafting then.

F_S2 force due to non-verticality of web at supports (the really nasty bits)

Good luck !

First off, I will look at the derivation of the effective length used for F_S2. Again, this is based on my interpretation of the commentary, and my maths ties up with the code equations, so I am fairly sure that I have worked it out correctly.

Figure 53 - plan view of deflection of strut with sprung ends

Figure 54 - isometric view of same beam showing opposite tilt of stiffeners

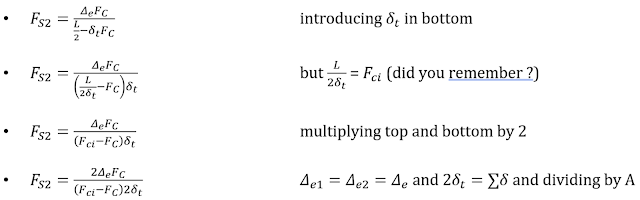
•       δ_t is the deflection under a unit load aka δ_e

•       Applying a force H, the deflection Δ = H . δ_t so H = Δ / δ_t

•       Aiming for :

      

(subscript e changed to t)

•       take moments about end: F . 2Δ = (Δ / δ_t) . L

• Which simplifies to F = L / (2 . δ_t)

•       Euler Buckling (relevance ?) F = ² . E . I_C / L_e²

•       Equating the forces (??)

•       L / (2 . δ_t) = ² . E . I_C / L_e²

•       L_e² = ² . E . I_C . 2δ_t / L

•        which is the stress at which the beam buckles

•       But let us treat it as a strut, similar but easier

•       Multiplying by area (of something) A gives the force F_ci

 

  •       Let us just ignore S =Z_pe/Z_xc as an annoyance

•       Which is the Euler buckling load leading us back to the Force F that would cause the rigid girder to spin on plan – this is not what most people think of as buckling, but …

•       i.e. F_ci = L / (2 . δ_t)

•       Please remember this

Figure 55 - combination of initial imperfection and flexural movement

But this is based on the assumption of a rigid strut with a high force (equal to the midspan force) applied at both ends. But these are simply supported beams with no moments/forces at the ends so the entire formula is extremely questionable (yes I am being polite again).

So not only is the force small, it is a complete nonsense and should not be applied.
Here endeth the nasty bit.

But what have they missed ?

As shown in the plan deflection plots, shown earlier, the forces where the U-frames push the top flange sideways will primarily push in one direction and the bearing stiffener provides the reaction at the end of the beam, in a similar way to the bearings at the end of the beam reacting to the vertical loads applied to the beam.

But I cannot see where this reaction is included. I would have thought that the maximum F_R force, plus reduced forces as the deflection reduces towards the bearing stiffeners, should be divided between the two bearing stiffeners – but that is just me.

Conclusion

U-frames and bearing stiffeners are a bit complicated but, like other bits in the code, clever people on committees have tried to find a way to capture very complex ideas in relatively simple equations (at least compared to non-linear finite element analysis).

The fact that you may have had no idea what was going on should not concern you since many engineers of not understand these things and, even with my experience, I may have made some errors in my assumptions above.

If, however, you can follow some of the above, at least how the U-frame stiffness is built up, together with the importance of plan bracing, then you will have made a start to becoming a thinking engineer, rather than someone who blindly plugs numbers into a formula and hopes that the formula is relevant. He more you can learn beyond this, the better you become.